Mathematical Modeling of a Class of Symmetrical Islamic Design

By: Mostafa Zahri

 

This video from Hafizan Halim is about how to draw a geometric pattern as an ornament from scratch.
Video source : How to draw a geometric pattern/ Youtube / Hafizan Halim

The art in the Islamic culture is very rich in spiritual and meditative messages. It uses complex geometric patterns and shapes. For instance, in wood for ornamental patterns, in bricks of buildings, in brass for decorations, paper, tiling, plaster, glass, etc. Islamic design can be found mainly in three distinct geometrical patterns, namely rectangular Kufic fonts, arabesque shapes, and polygonal designs.

The largest class of Islamic design employs, in general, complex and symmetrical polygons, where, in each polygonal graph, the starting design is a cell in the form of a symmetrical shape. The creation of the symmetrical rectangular, triangular, and circular patterns leads to beautiful ornamental patterns.

The mathematical modeling of these cells is based on iterative techniques for generating beautiful and colored patterns. Different methods were used for seeking explicit relationships between a simple single cell and the corresponding complex multiplicity, either for constructing symmetrical groups for infinite periodic patterns form the 17 classes of the two-dimensional crystallographic groups or by using planar geometrical transformations, translations, rotations, reflections, and glide reflections for rearranging and space filling.

The modeling of symmetries in nature and sciences brought together the interest of many scientists for developing several techniques. In the authors used the logistic mapping for generating and describing pictures created by merging symmetry and chaos.

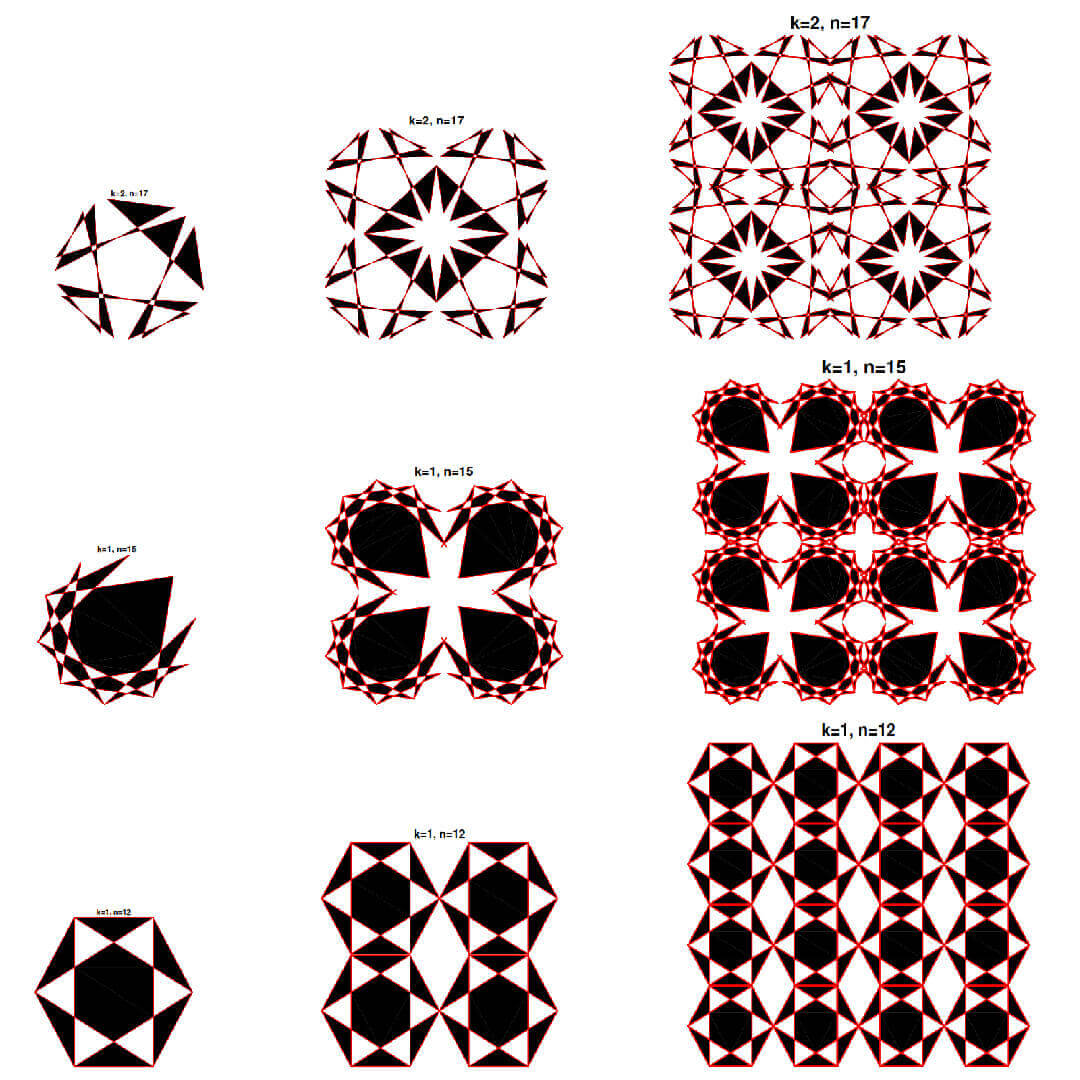
In, we studied the existence and the symmetry of the multiple barycenters, especially in the unit circle considered as one-dimensional manifold. The generated vertices are, for special values of n, the so-called multiple barycenters on S 1.

Moreover, since mapping circular patterns on curved surfaces in a three-dimensional space is in general a nontrivial task we propose a new algorithm for generating planar and isometric patterns. We construct and characterize vertices on the unit circle similar to the multiple barycenters using repeated iterations of emerged numbers.

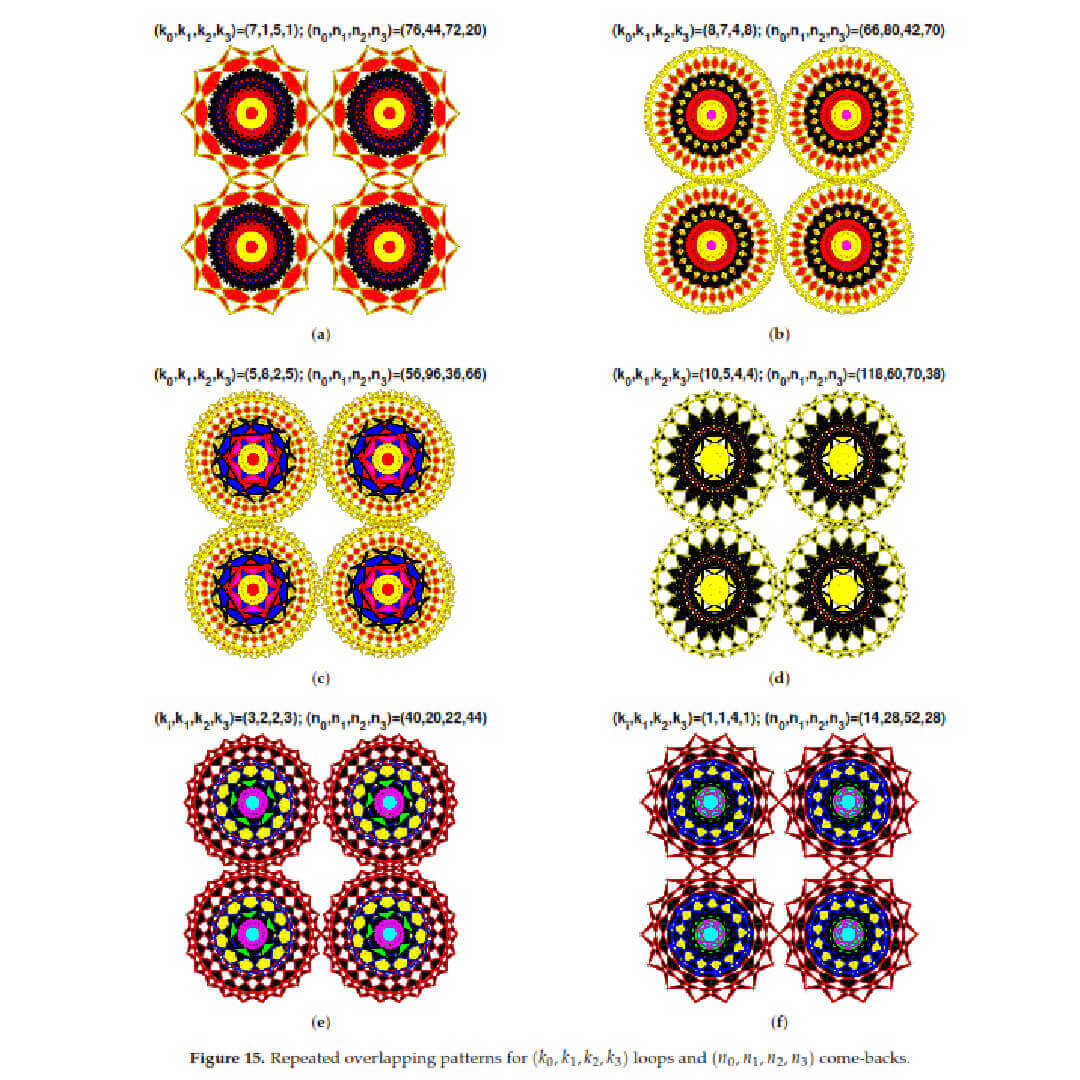
Thereafter, connecting these barycenters as vertices with finite number of edges describes and classifies special periodic sequences. These sequences are the main source for constructing symmetrical designs, especially Islamic design.