Flat-Foldable Planar
Freeform Rigid-Foldable Structure using Bidirectionally Flat-Foldable Planar Quadrilateral Mesh
Tomohiro Tachi
The University of Tokyo
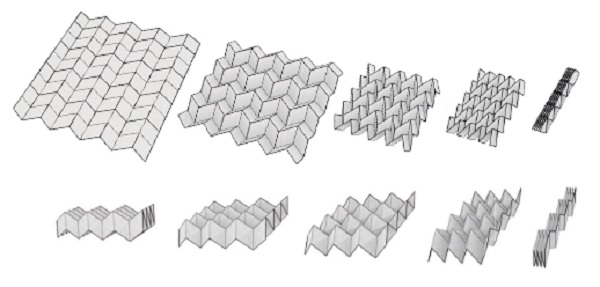
Figure 1: Folding motions of Miura-ori (Top) and eggbox pattern (Bottom).
This paper presents a computational design method to obtain collapsible variations of rigid-foldable surfaces, i.e., continuously and finitely transformable polyhedral surfaces, homeomorphic to disks and cylinders.
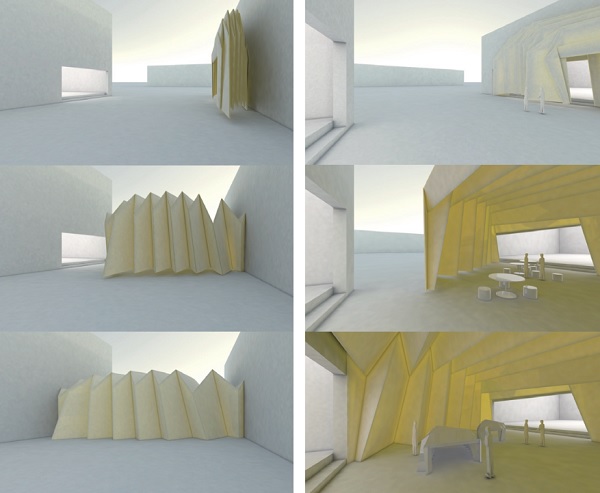
Figure 2: A corrugated vault used as a transformable architecture that connects two existing buildings.
Two novel techniques are proposed to design such surfaces: a technique for obtaining a freeform variation of a rigid-foldable and bidirectionally flat-foldable disk surface, which is a hybrid of generalized Miura-ori and eggbox patterns, and a technique to generalize the geometry of cylindrical surface using bidirectionally flat-foldable planar quadrilateral mesh by introducing additional constraints to keep the topology maintained throughout the continuous transformation.
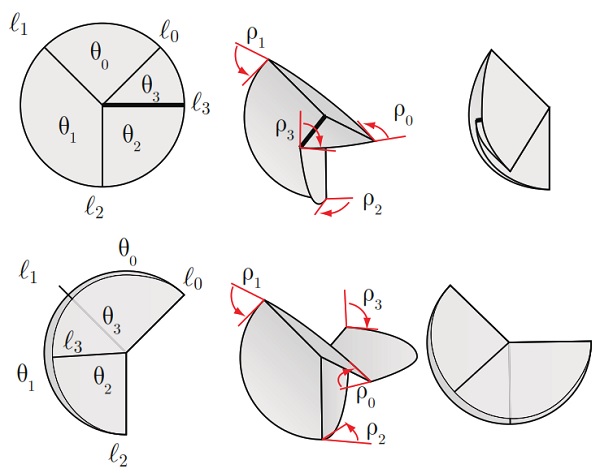
Figure 3: The folding motions of vertices of origami (Top) and discrete Voss surface (Bottom). Note that set of planes used is identical.
Proposed methods produce freeform variations of rigid-foldable structures that have not been realized thus far. Such a structure forms a one-DOF mechanism with two possible flat states. This enables the designs of deployable structures useful for packaging the boundary of architectural spaces, space structures, and so on.
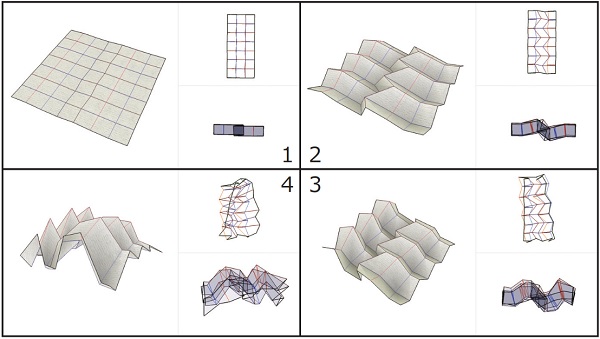
Figure 4: The design process of hybrid pattern obtained by deforming a square grid. Red and dark blue lines indicate mountain and valley foldlines, and pink and light blue indicate complementary mountain and valley foldlines. Each screen shows 3D configuration (left) developed state (upper-right) and flat-foldable shape (lowerright)
A polyhedral surface composed of rigid facets connected by rotational edges forms a kinetic mechanism: a rigid folding mechanism. Several collapsible structures are proposed using this type of kinetic mechanism, such as Miura-ori [Miura 1980] and eggbox patterns.

Figure 5: The folding motion of a generalized eggbox pattern.
In order to apply such kinetic surfaces to design purposes, it is required that we can control the three-dimensional shape of the surface and its behavior so that they are consistent with the design requirements.
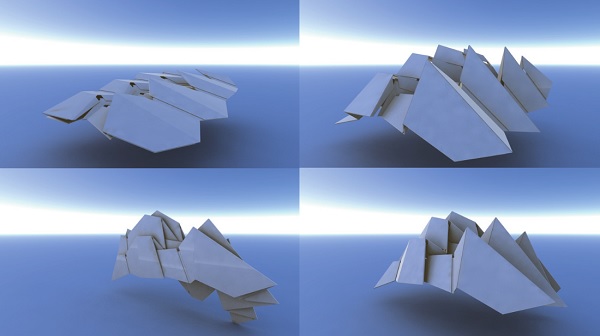
Figure 6: The folding motion of hybrid BDFFPQ mesh.
Design requirements in architectural context include environmental conditions, functional requirements, configuration of surrounding buildings and structures, and user’s preference.

Figure 7: A closed strip of facets (Left). A loop can continuously shrink or expand using conditions around vertices (Right).
However, since the rigid-foldability of known surfaces strongly relies on the symmetry of the pattern, designing such structures could not be done in an ad-hoc approach; the geometric constraints must be generally investigated to solve an inverse problem of obtaining a pattern from the resulting form and behavior.
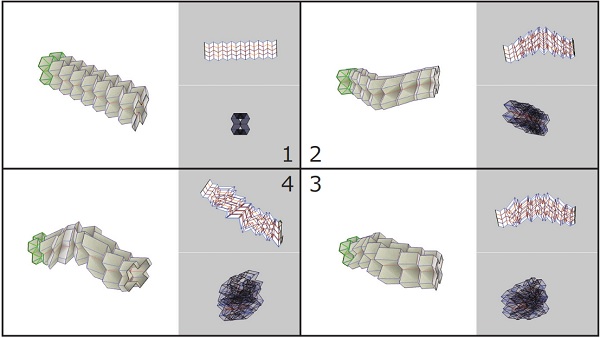
Figure 8: A design process of rigid-foldable cylinder. The initial state is a cylinder by [Tachi 2009b]. The surface is deformed keeping the condition of BDFFPQ mesh while a loop strip (two strips in this redundant case) is maintained to be rigid, as indicated by green rigid bars.
The objective of our study is thus to freely design kinetic forms using design methods based on computational geometry.

Figure 9: A crease pattern of generalized cylinder.
As the first step to achieve this goal, the author proposed a freeform design method of kinetic structures based on origami (developable surface) to provide design variations of Miura-ori through an interactive design system in which a user can deform the surface freely while sustaining the rigid-foldability of the surface ([Tachi 2009a]).

Figure 10: Folded model of a rigid-foldable cylinder. Left: in the developed state, Middle:Intermediate state, Right: flat-folded state.
This method yields variations of rigid-foldable origami models that have never been achieved otherwise, however, the method was not general enough for design applications, especially of architecture, since the developability condition used is not always an essential condition when we construct structures from multiple parts.
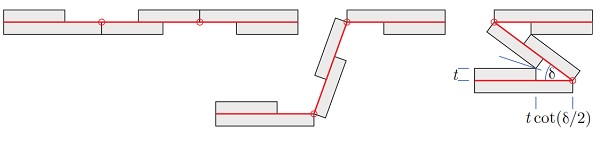
Figure 11: Thickening using two layers of constant thickness panels.
In particular, this developability condition disables the design of rigidfoldable collapsible cylinders, realization of which can contribute the designs of surrounding of a volume of a space, collapsible containers, compound surface structures, and so on. This limitation mainly comes from the fact that a cylindrical surface cannot have a globally developed state under the conventional definition.
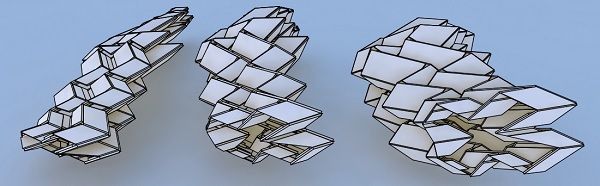
Figure 12: Cylindrical rigid-foldable BDFFPQ mesh consisting of thick panels.
This paper provides a novel method that enables a freeform variation of such rigidfoldable structure, not restricted by the developability of the surface or the disk topology. We will solve this design problem by extending and combining the ideas of generalized Miura-ori [Tachi 2009a] (or flat-foldable 4-valent-vertex origami) and generalized eggbox pattern (or discrete Voss surface) [Schief et al. 2007] to produce bidirectionally flat-foldable planar quadrilateral mesh.
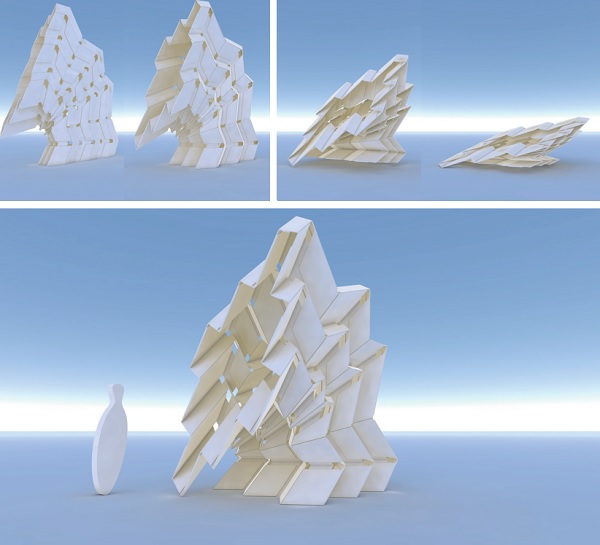
Figure 13: Folding motion of cylindrical structure using thick panels.
Such a mesh has nice generalized characteristics: it is bidirectionally flat-foldable and produces one-DOF mechanism. Required design condition is loose compared to purely developable surface and it allows asymmetric and cylindrical variations. We will show a novel design method to obtain rigid-foldable collapsible cylinders by deforming symmetric rigid-foldable cylinders of [Tachi 2009b] into asymmetric ones.



























Comments