Auxetic Surface
Bistable Auxetic Surface Structures
IAN CHEN1, JULIAN PANETTA2, MAX SCHNAUBELT3, MARK PAULY1
1EPFL, Switzerland
2UC Davis, United States
3TUM, Germany
We present Bistable Auxetic Surface Structures, a novel deployable material system based on optimized bistable auxetic cells.
Such a structure can be flat-fabricated from elastic sheet material, then deployed towards a desired double-curved target shape by activating the bistable mechanism of its component cells.
A unique feature is that the deployed model is by design in a stable state. This facilitates deployment without the need of complex external supports or boundary constraints.
 We introduce a computational solution for the inverse design of our Bistable Auxetic Surface Structures. Our algorithm first precomputes a library of bistable auxetic cells to cover a range of in-plane expansion/contraction ratios, while maximizing the bistability and stiffness of the cell to ensure robust deployment.
We introduce a computational solution for the inverse design of our Bistable Auxetic Surface Structures. Our algorithm first precomputes a library of bistable auxetic cells to cover a range of in-plane expansion/contraction ratios, while maximizing the bistability and stiffness of the cell to ensure robust deployment.
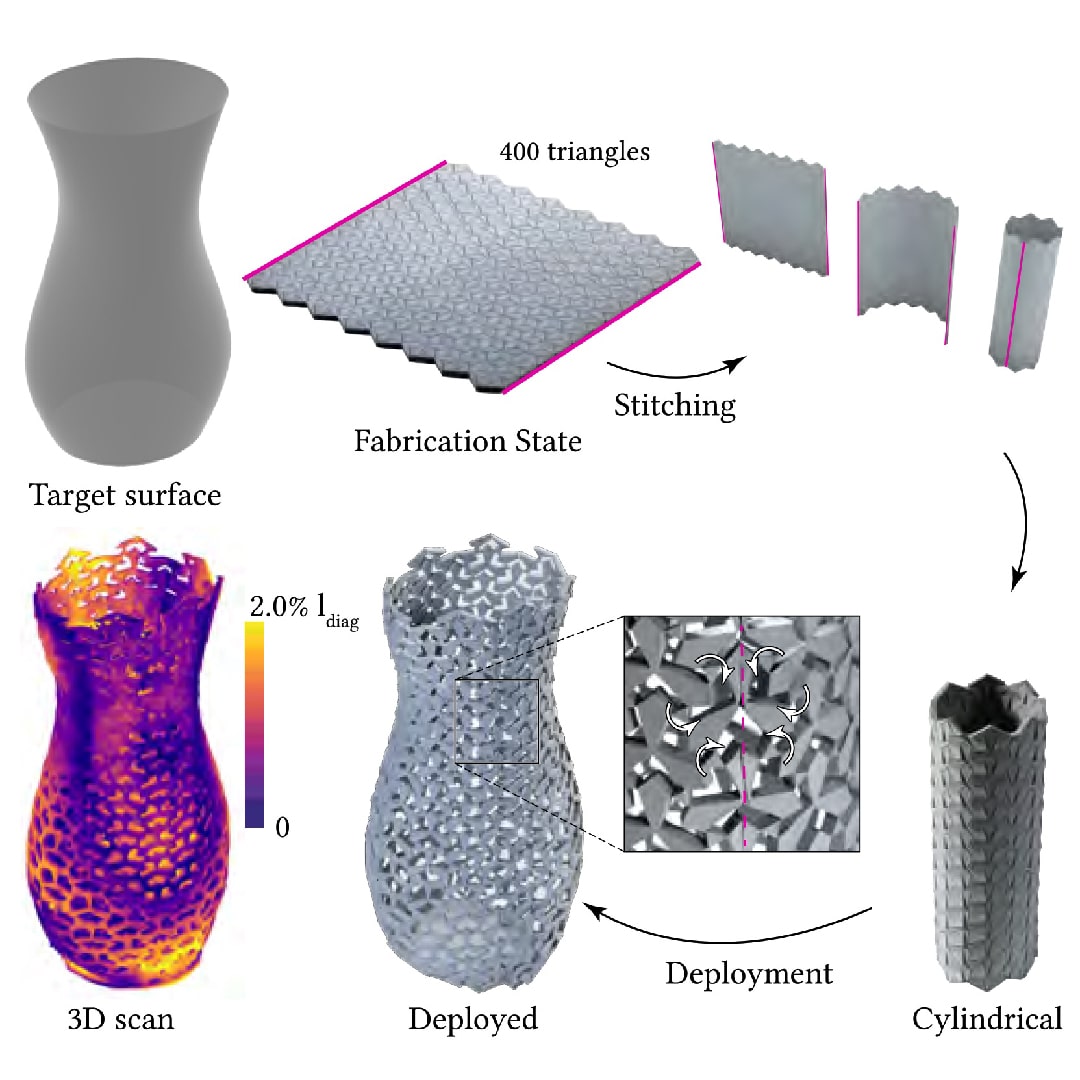
 We then use metric distortion analysis of the target surface to compute the planar fabrication state as a composition of cells that best matches the desired deployment deformation.
We then use metric distortion analysis of the target surface to compute the planar fabrication state as a composition of cells that best matches the desired deployment deformation.
As each cell expands or contracts during deployment, metric frustration forces the surface towards its target equilibrium state. We validate our method with several physical prototypes.
 Large-scale shape transformation is crucial in many applications across different length scales, ranging from encapsulation and release of medicine to the construction of space structures.
Large-scale shape transformation is crucial in many applications across different length scales, ranging from encapsulation and release of medicine to the construction of space structures.
In particular, structures that can deploy from a flat state to a desired target geometry can significantly reduce the time and cost of fabrication, transport, and construction.
 In extreme environments such as outer space or the deep sea, deployable structures are often the only feasible means of erection.
In extreme environments such as outer space or the deep sea, deployable structures are often the only feasible means of erection.
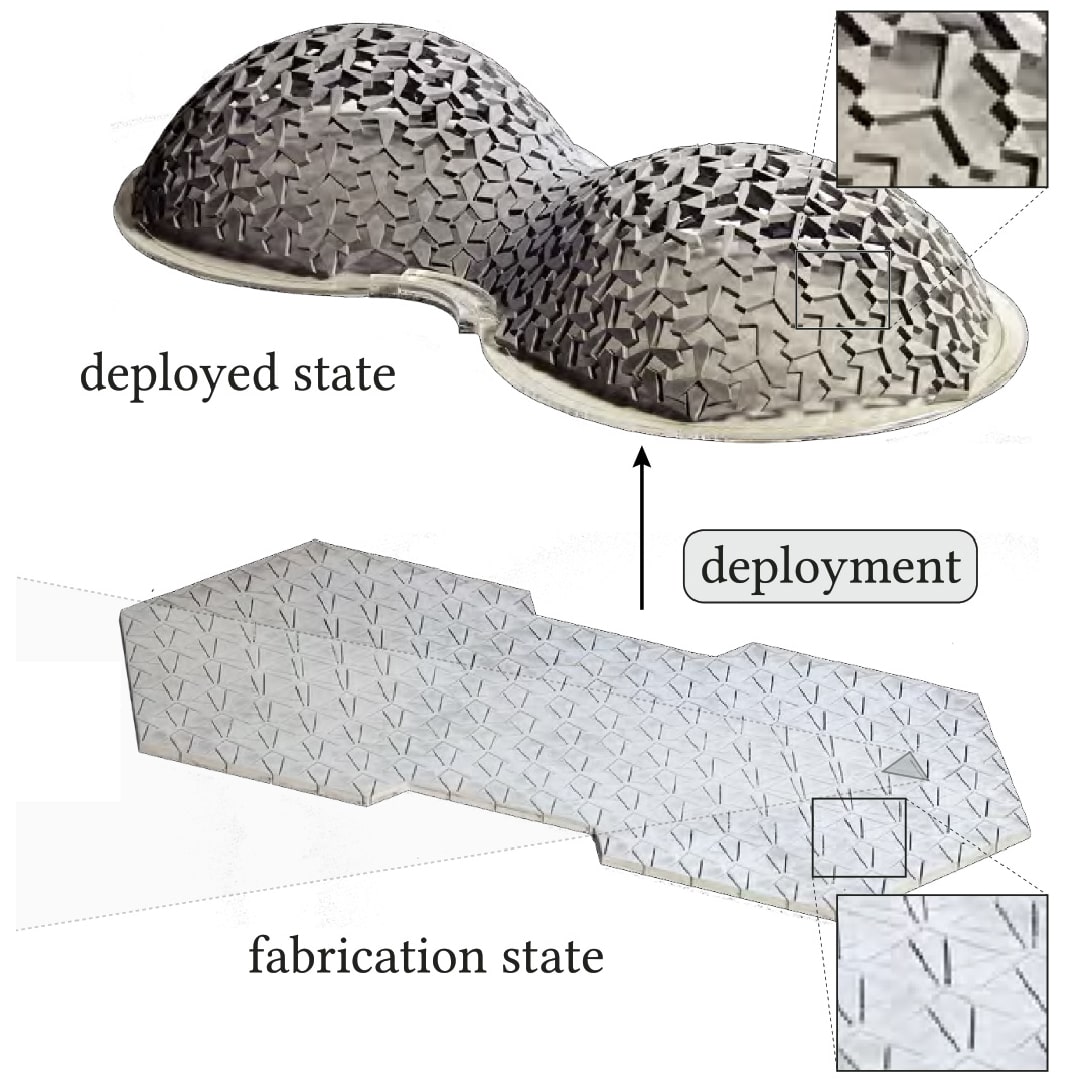 Traditional deployable structures often employ regular periodic patterns to simplify fabrication and achieve deployment through the application of specifically designed boundary constraints.
Traditional deployable structures often employ regular periodic patterns to simplify fabrication and achieve deployment through the application of specifically designed boundary constraints.
Examples of this type of structures include grid shells, cable nets, inflatable domes or deployable solar panels. Shape control through boundary constraints is inherently limited, however, and typically leads to a narrow range of possible shapes.
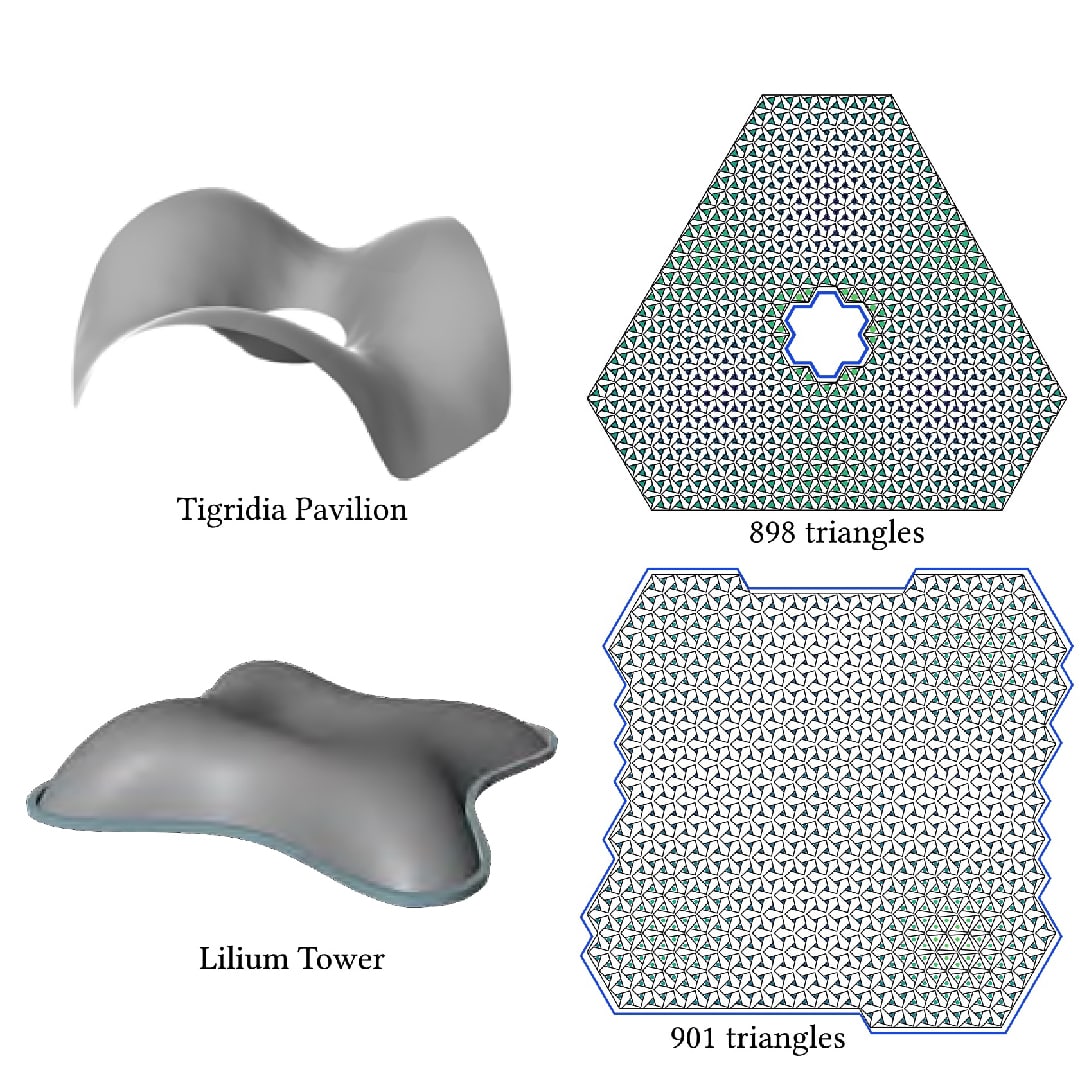 To achieve more complex target shapes, recent work has leveraged advanced manufacturing technology to embed knowledge of the target shape into the material system itself.
To achieve more complex target shapes, recent work has leveraged advanced manufacturing technology to embed knowledge of the target shape into the material system itself.
This shifts the complexity from the boundary to the interior of the structure and often enables a simplified, global deployment. Researchers have also incorporated smart materials that react to certain environmental conditions to achieve autonomous shape change.
Typically, smart materials such as shape memory polymers, liquid crystal elastomers and artificial hydrogels are fabricated in one state, and when exposed to a trigger, transform to a second state.
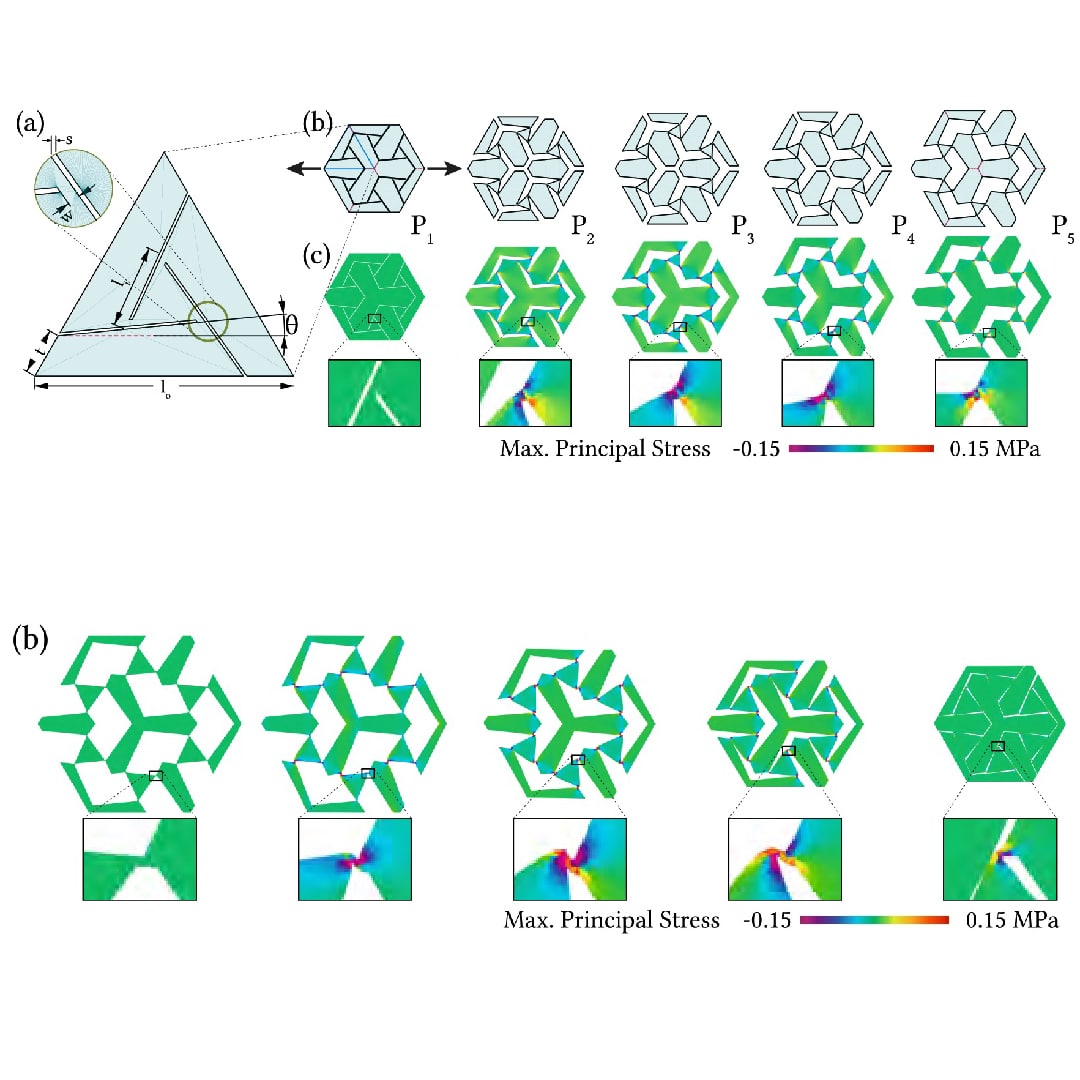 Contributions. In our work we propose a novel mechanics-based solution to achieve stable shape transformation and deployment. We leverage two key principles to solve this challenging problem, namely (i) deployment of a planar surface by spatially varying isotropic expansion, and (ii) auxeticity with tunable mechanical bistability.
Contributions. In our work we propose a novel mechanics-based solution to achieve stable shape transformation and deployment. We leverage two key principles to solve this challenging problem, namely (i) deployment of a planar surface by spatially varying isotropic expansion, and (ii) auxeticity with tunable mechanical bistability.
We introduce a parameterized bistable cell that offers a continuous range of extensibility at a second stable equilibrium state through geometric variation.
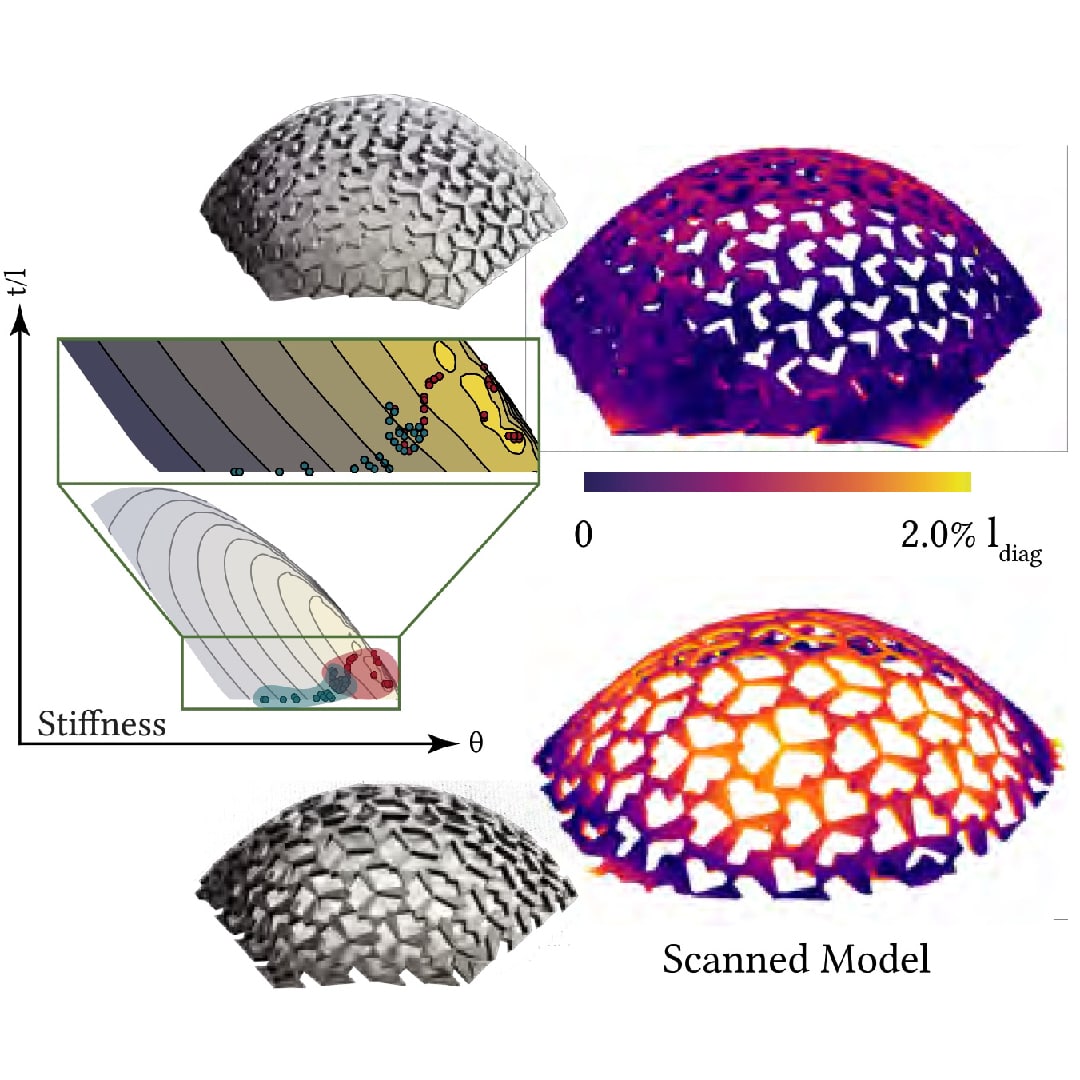
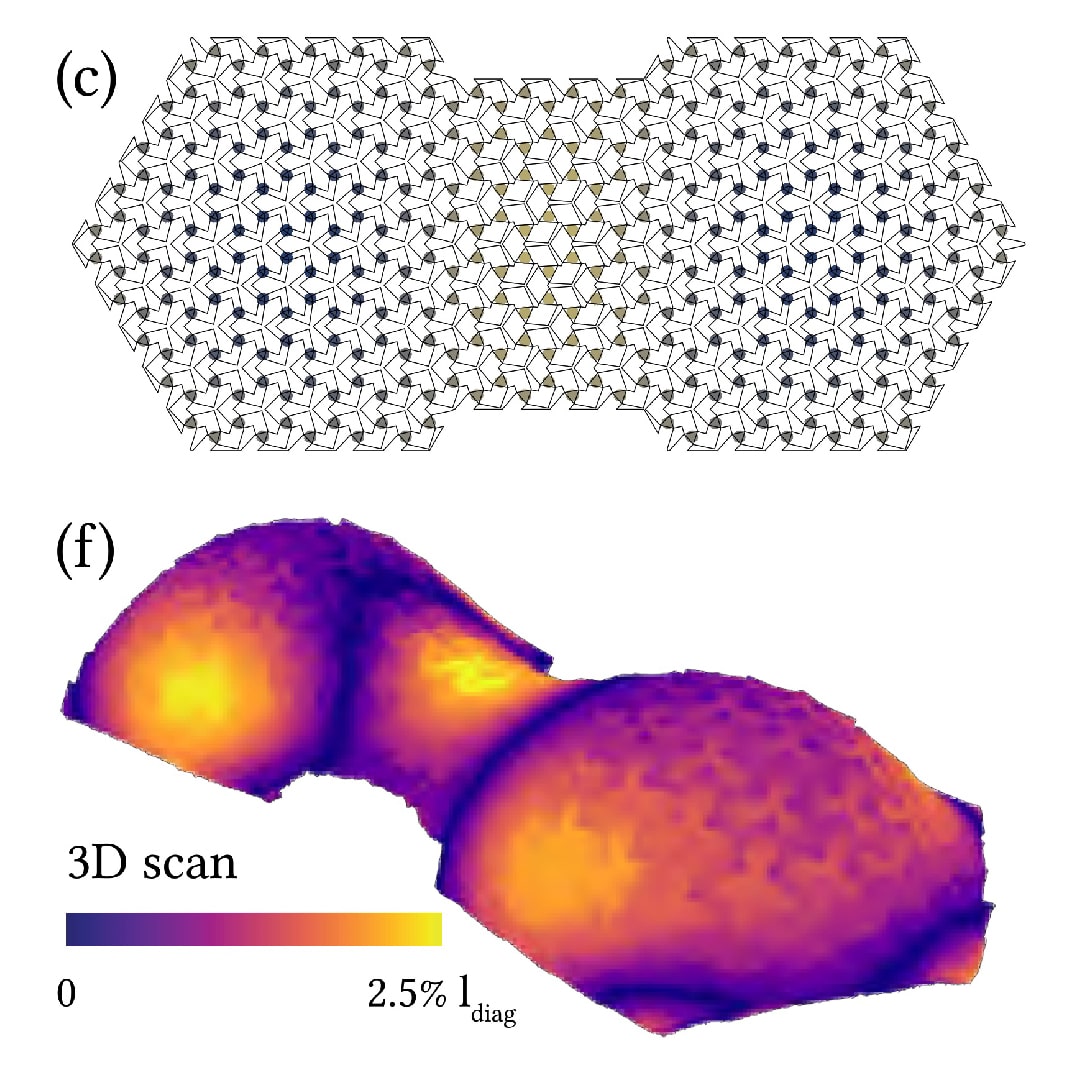
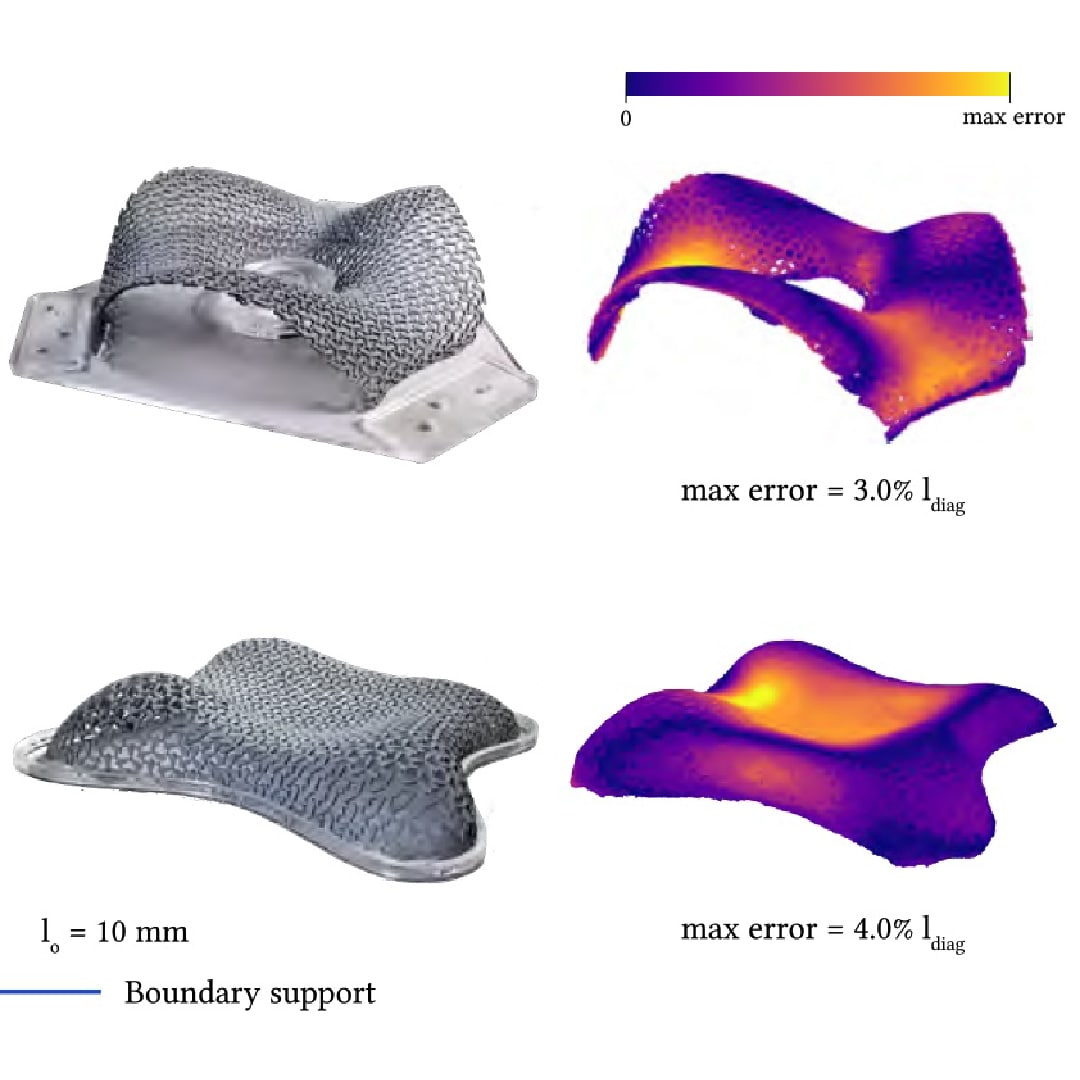
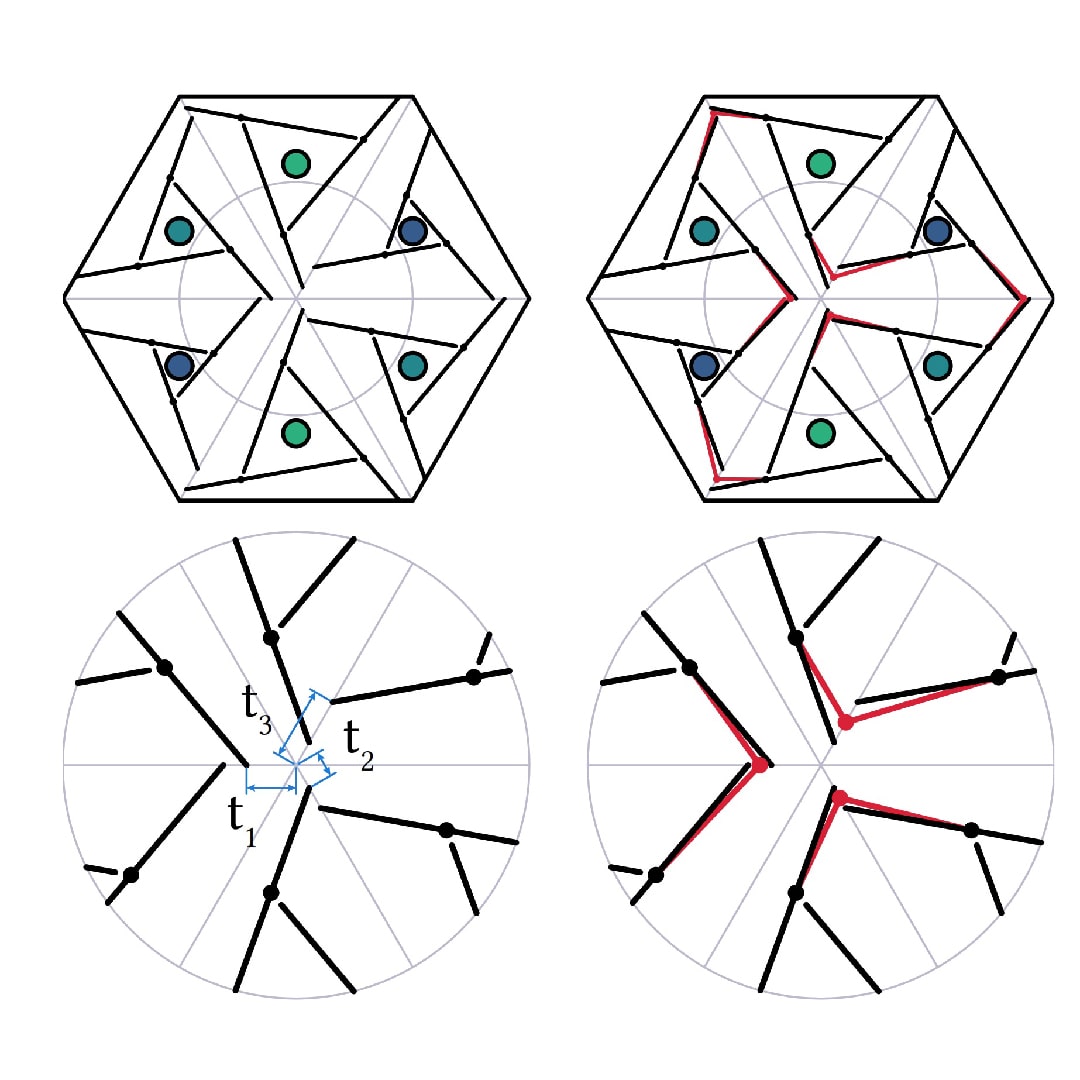 We show how a tiling of such cells can be optimized to program the required metric frustration into a planar sheet such that the structure globally deploys into a desired target surface and remains stable after deployment forces are removed. Our computational method is validated through scanned physical prototypes and mechanical testing.
We show how a tiling of such cells can be optimized to program the required metric frustration into a planar sheet such that the structure globally deploys into a desired target surface and remains stable after deployment forces are removed. Our computational method is validated through scanned physical prototypes and mechanical testing.



























Comments